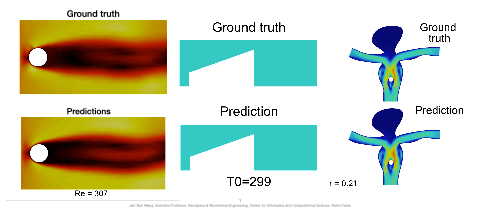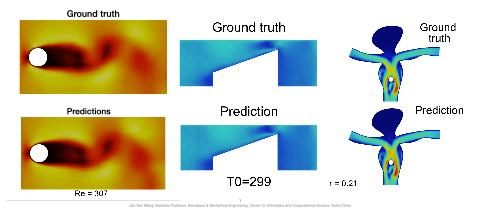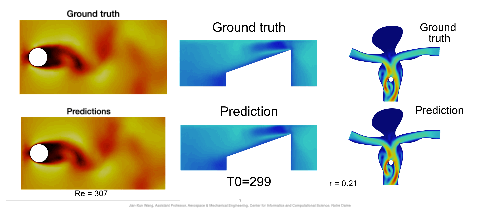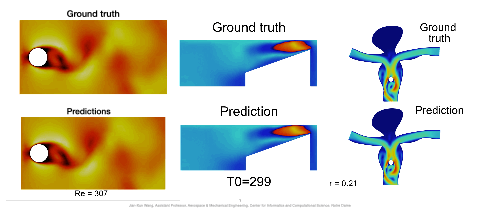
We are welcoming submission of original research to an article collection entitled “Physics-informed Machine Learning and Its Real-world Applications” of Scientific Reports (Springer Nature). As Guest Editor for the Collection, I hope you will consider this excellent outlet for your research in this field.
This article collection is a great opportunity to highlight this important area, and we hope you will be able to contribute. Please don’t hesitate to contact me if you have any questions. The deadline is Nov. 30, 2022. For more details, please see https://www.nature.com/collections/hdjhcifhad/guest-editors